Modelación de la viscosidad dinámica de fluidos newtonianos a partir de la teoría de Eyring y la energía libre de Helmholtz residual Modeling the dynamic viscosity of Newtonian Fluids using the Eyring’s Theory and the Residual Helmholtz Free Energy

Resumen

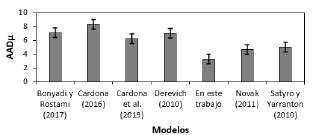
En este trabajo se modela la viscosidad dinámica de fluidos newtonianos a partir de la teoría de Eyring junto con la energía libre de Helmholtz residual. Para la estimación de esta energía se utiliza una versión modificada de la ecuación cúbica de estado de Peng-Robinson. Los parámetros ajustables del modelo se han determinado a partir de datos experimentales en la zona coexistencia líquido-vapor para n-alcanos y n-alcoholes. Posteriormente estos parámetros se han generalizado utilizando expresiones matemáticas simples que dependen del peso molecular de cada sustancia. Se evalúan las capacidades predictivas del modelo en condiciones de una sola fase. Las desviaciones absolutas durante el proceso de correlación son menores de 3,27%, mientras que en el proceso de predicción son menores de 5,60%. El modelo generalizado es extendido a mezclas binarias utilizando una regla de mezcla simple sin y con coeficientes de interacción, con desviaciones absolutas de 8,19% y 3,45%, respectivamente. Finalmente, el modelo es comparado con otros en la literatura y los resultados estadísticos muestran que proporciona resultados aceptables.